Difference between revisions of "Sandbox"

From WikiManual
Jump to: navigation, search
(math markup .... NOT! ;))
Line 19: Line 19:
  
 
Darwinbots is not spelled with a z.  That is, Darwinbotz is most, most wrong.
 
Darwinbots is not spelled with a z.  That is, Darwinbotz is most, most wrong.
 +
 +
===Geometry===
 +
 +
:testing to see if this wiki supports Math Markup.
 +
:apparently not.
 +
:was looking to display it as it is at http://en.wikipedia.org/wiki/Pi#Geometry
 +
:too bad ... this would give us an easy way to diplay math/formulas, etc.
 +
:Nums ...
 +
:i think there is a way to impliment this ...
 +
:perhaps along the lines of implimenting the file upload thing. {{User:Griz/sig}}
 +
 +
 +
<math>\pi</math> appears in many formulae in [[geometry]] involving [[circle]]s and [[sphere]]s.
 +
 +
{| border="1" cellspacing="4" cellpadding="4" style="border-collapse: collapse;"
 +
!Geometrical shape
 +
!Formula
 +
|-
 +
|[[Circumference]] of circle of [[radius]] ''r'' and [[diameter]] ''d''
 +
|<math>C = \pi d = 2 \pi r \,\!</math>
 +
|-
 +
|[[area (geometry)|Area]] of circle of radius ''r''
 +
|<math>A = \pi r^2 \,\!</math>
 +
|-
 +
|Area of [[ellipse]] with semiaxes ''a'' and ''b''
 +
|<math>A = \pi a b \,\!</math>
 +
|-
 +
|[[Volume]] of sphere of radius ''r'' and diameter ''d''
 +
|<math>V = \frac{4}{3} \pi r^3 = \frac{1}{6} \pi d^3 \,\!</math>
 +
|-
 +
|[[Surface area]] of sphere of radius ''r''
 +
|<math>A = 4 \pi r^2 \,\!</math>
 +
|-
 +
|Volume of [[cylinder]] of height ''h'' and radius ''r''
 +
|<math>V = \pi r^2 h \,\!</math>
 +
|-
 +
|Surface area of cylinder of height ''h'' and radius ''r''
 +
|<math>A = 2 ( \pi r^2 ) + ( 2 \pi r ) h = 2 \pi r (r + h) \,\!</math>
 +
|-
 +
|Volume of [[cone]] of height ''h'' and radius ''r''
 +
|<math>V = \frac{1}{3} \pi r^2 h \,\!</math>
 +
|-
 +
|Surface area of cone of height ''h'' and radius ''r''
 +
|<math>A = \pi r \sqrt{r^2 + h^2} + \pi r^2 =  \pi r (r + \sqrt{r^2 + h^2}) \,\!</math>
 +
|}
 +
 +
(All of these are a consequence of the first one, as the area of a circle can be written as
 +
''A'' = &int;(2''&pi;r'')d''r'' ("sum of [[annulus|annuli]] of infinitesimal width"), and others concern a surface or [[solid of revolution]].)
 +
 +

Revision as of 12:58, 21 November 2005

a sandbox to play in ...

practice wiki code and formatting here ...
ask questions, put demo code ... whatever.
remember ... you can't hurt anything ... all pages can be reverted to what they were
and all page changes are kept in history. Click the historytab above to view them. Griztalk

Darwinbots is not:

(Inspired by "Wikipedia is not...")

Darwinbots is not a pizza.

Darwinbots is not a provelactic.

Darwinbots is not a middle name.

Darwinbots is not an essential part of a balanced breakfast.

Darwinbots is not spelled with a z. That is, Darwinbotz is most, most wrong.

Geometry

testing to see if this wiki supports Math Markup.
apparently not.
was looking to display it as it is at http://en.wikipedia.org/wiki/Pi#Geometry
too bad ... this would give us an easy way to diplay math/formulas, etc.
Nums ...
i think there is a way to impliment this ...
perhaps along the lines of implimenting the file upload thing. Griztalk


\pi appears in many formulae in geometry involving circles and spheres.

Geometrical shape Formula
Circumference of circle of radius r and diameter d 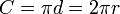C = \pi d = 2 \pi r \,\!
Area of circle of radius r A = \pi r^2 \,\!
Area of ellipse with semiaxes a and b A = \pi a b \,\!
Volume of sphere of radius r and diameter d 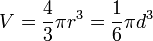V = \frac{4}{3} \pi r^3 = \frac{1}{6} \pi d^3 \,\!
Surface area of sphere of radius r 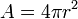A = 4 \pi r^2 \,\!
Volume of cylinder of height h and radius r V = \pi r^2 h \,\!
Surface area of cylinder of height h and radius r 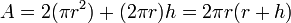A = 2 ( \pi r^2 ) + ( 2 \pi r ) h = 2 \pi r (r + h) \,\!
Volume of cone of height h and radius r V = \frac{1}{3} \pi r^2 h \,\!
Surface area of cone of height h and radius r 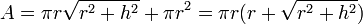A = \pi r \sqrt{r^2 + h^2} + \pi r^2 = \pi r (r + \sqrt{r^2 + h^2}) \,\!

(All of these are a consequence of the first one, as the area of a circle can be written as A = ∫(2πr)dr ("sum of annuli of infinitesimal width"), and others concern a surface or solid of revolution.)

Also, the angledegrees) is equal to π radians.

Retrieved from "http://wiki.darwinbots.com/index.php?title=Sandbox&oldid=1268"